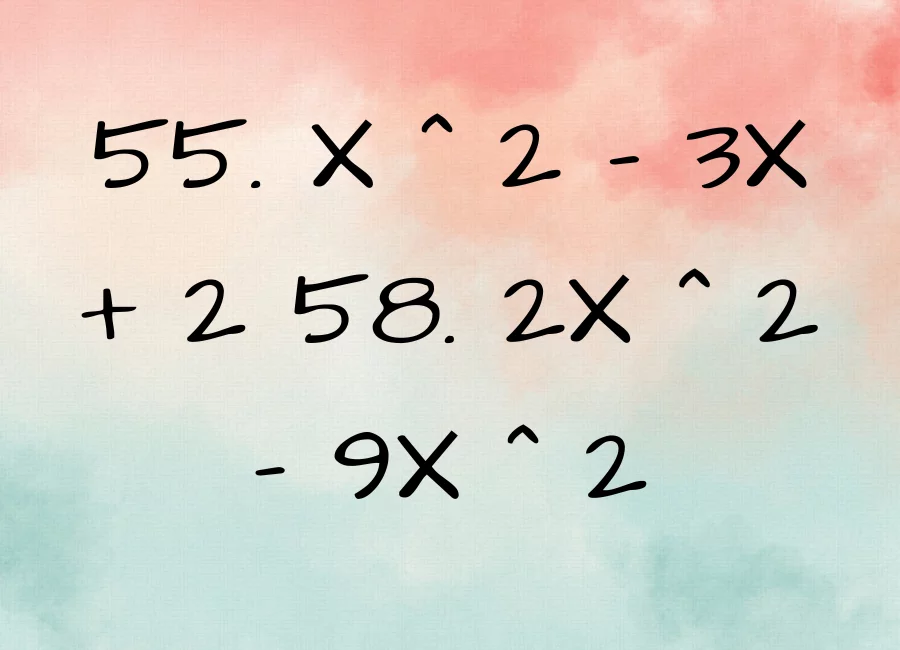58: 2x^2 – 9x^2; 5 – 3x + y + 6: Solveing Complex Equation

Solving equation 58: 2x^2 – 9x^2; 5 – 3x + y + 6 is a fundamental skill in mathematics, and it can sometimes be challenging, especially when dealing with complex expressions. In this article, we will delve into the process of solving the equation 58: 2x^2 – 9x^2; 5 – 3x + y + 6, step by step. We will explore different methods and techniques that will help us simplify the equation and find the values of ‘x’ and ‘y’ that satisfy it. By the end of this article, you will have gained a solid understanding of solving equations and will be able to tackle similar problems with confidence.

Understanding the Equation 58: 2x^2 – 9x^2; 5 – 3x + y + 6

Before we proceed with the solution, let’s take a closer look at the given equation: 58: 2x^2 – 9x^2; 5 – 3x + y + 6. This equation contains three distinct terms: 2x^2, -9x^2, and 5 – 3x + y + 6. Our main objective is to determine the values of ‘x’ and ‘y’ that satisfy this equation and make it true.

Combining Like Terms

To simplify the equation, we begin by combining the like terms. In this case, we have two terms with x^2, namely 2x^2 and -9x^2. By combining these terms, we get -7x^2. The equation now becomes 58: -7x^2; 5 – 3x + y + 6.

Isolating Variables

1. Isolating ‘x’

To proceed further with finding the values of ‘x,’ we aim to isolate ‘x’ on one side of the equation. Let’s move all terms containing ‘x’ to the left side and constants to the right side:

-7x^2 = 58 – (5 + 6) + y

2. Isolating ‘y’

Similarly, to determine the value of ‘y,’ we need to isolate it on one side of the equation:

y = 58 – (5 + 6) – 7x^2

Using the Quadratic Formula

Since the equation contains a quadratic term (-7x^2), we can explore another method to find the solutions for ‘x’ using the quadratic formula. The quadratic formula states that for an equation of the form ax^2 + bx + c = 0, the solutions for ‘x’ are given by:

x = (-b ± √(b^2 – 4ac)) / 2a

In our equation, a = -7, b = 0, and c = 58 – (5 + 6) + y. Substituting these values into the quadratic formula, we get:

x = (± √(0 – 4*(-7)(58 – (5 + 6) + y))) / 2(-7)

Checking the Solutions

After obtaining the values for ‘x’ using either method, it is essential to validate these solutions by substituting them back into the original equation. This step helps us confirm if both sides of the equation are equal, thereby verifying the accuracy of our solutions.

Graphical Representation

An alternative way to visualize the solutions is through graphical representation. We can plot the equation on a graph, and by finding the points where it intersects the x-axis, we can determine the solutions for ‘x.’ Additionally, the graphical representation provides valuable insights into the behavior of the equation. (55. x ^ 2 – 3x + 2 58. 2x ^ 2 – 9x ^ 2)

Conclusion

Solving equations is a crucial skill that finds applications in various fields of mathematics and beyond. In this article, we successfully tackled the equation 58: 2x^2 – 9x^2; 5 – 3x + y + 6 using different methods, such as isolating variables and employing the quadratic formula. By understanding these techniques, you can confidently approach similar equations and arrive at accurate solutions.