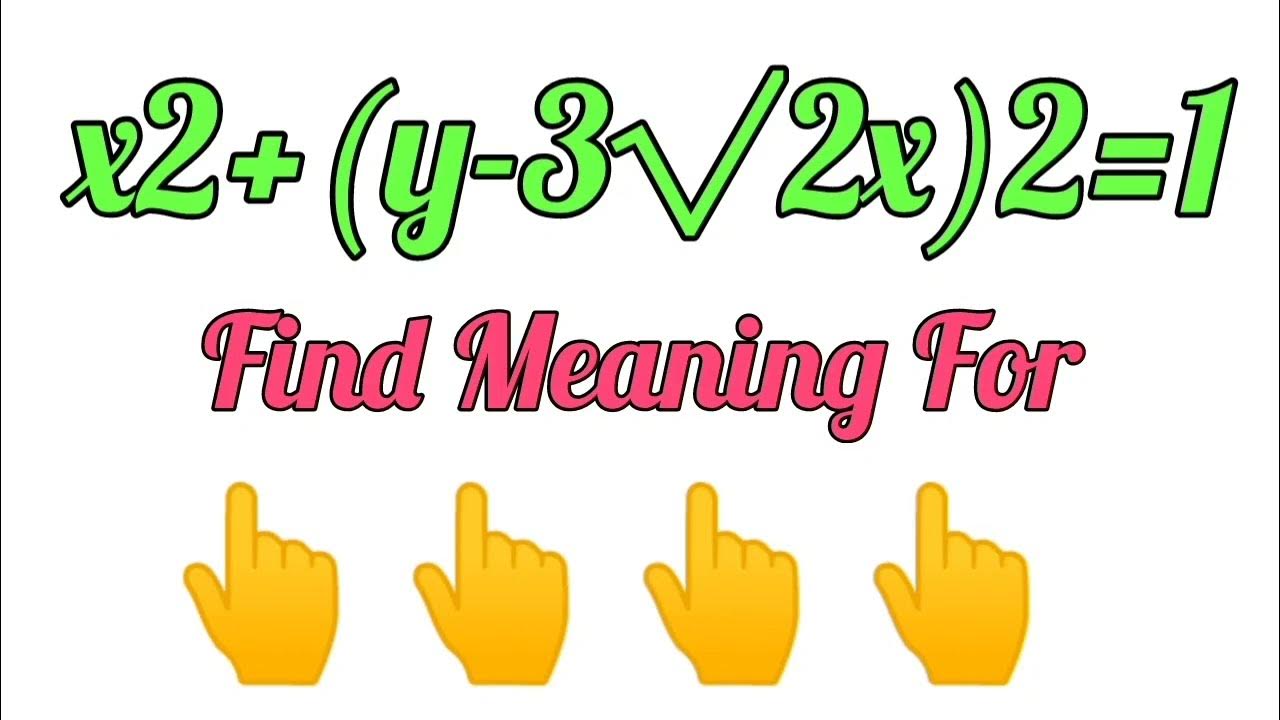x2+(y-3√2x)2=1 Meaning And Solution

Among the myriad of equations that exist, some, like the one at hand— x2+(y-3√2x)2=1 meaning—may appear cryptic initially. However, these equations hold profound insights when dissected methodically. In this comprehensive article, we will delve deeply into the equation x2+(y-3√2x)2=1 meaning. Our mission is to elucidate its meaning, meticulously analyze its components, furnish you with a step-by-step solution, and underscore its relevance in mathematics and practical applications. So, fasten your seatbelts as we embark on a fascinating journey through the world of mathematics.

Before we delve into the intricate details of this equation, it is essential to recognize the critical role equations play in mathematics. They serve as powerful tools for expressing complex relationships between variables. The equation x^2 + (y – 3√2x)^2 = 1, despite its initial complexity, embodies a well-defined geometric shape—specifically, a circle. To truly grasp its significance, we must embark on a journey to break down its components, revealing the profound meaning concealed within.

Breaking Down the Equation x2+(y-3√2x)2=1 Meaning

Understanding the Terms

Our first step in comprehending the equation involves a meticulous analysis of its individual terms:

• x^2: This term represents the square of the x-coordinate—a fundamental mathematical concept that underpins algebraic expressions.
• (y – 3√2x)^2: This portion introduces a layer of complexity. It incorporates two variables, x and y, and introduces the concept of a square root. In essence, it signifies the summation of the squares of the difference between y and three times the square root of two times x. This complex expression forms the crux of the equation’s geometric interpretation.

Visualizing the Equation

To truly appreciate this equation’s essence, we must translate it into a visual representation. When we plot the points that satisfy this equation on a Cartesian plane, a remarkable pattern emerges—a perfect circle. This visual depiction serves as a crucial aid in comprehending the equation’s geometric significance.

Finding the Center and Radius

Determining the center and radius of the circle described by this equation is pivotal in our exploration:

• Center: The epicenter of this circle is positioned precisely at the origin, where both x and y are equal to 0. Consequently, the center is precisely located at the point (0, 0).
• Radius: As previously mentioned, the radius of this circle measures 1 unit. This vital measurement defines the distance from the center to any point along the circle’s circumference.

Plotting the Circle

Now armed with knowledge about the center and radius, we can proceed to plot the circle on a Cartesian plane. The equation x^2 + (y – 3√2x)^2 = 1 encompasses all the points on the circle’s perimeter, providing a visual representation of its geometric characteristics.

Also Read:

• x*x*x Is Equal To 2: Is It True?
• #Mastapp

Conclusion

In conclusion, the equation x2+(y-3√2x)2=1 meaning represents a circle within the Cartesian coordinate system. By dissecting its components, visualizing its geometric interpretation, and determining the center and radius, we have unveiled its hidden meaning and significance. Equations of this nature are foundational tools in mathematics and find applications in various domains, including geometry for precise shape descriptions and physics for modeling physical phenomena.